Complete Elliptic Integral of the Second Kind

Online calculator Perimeter of an ellips

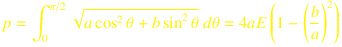
$p = \int_0^{\pi / 2} \sqrt{a \cos^2 \theta + b \sin^2 \theta}\ d \theta = 4 a E\left(1 - \left(\frac{b}{a}\right)^2\right)$
E(m) is the complete elliptic integral of the second kind with parameter m = k²

Jan. 18 2023
Takayuki HOSODA

Japanese edition is here.

Perimeter of an ellips

The calculation is performed using the Complete Elliptic Integrals of the Second Kind.
The approximation of Ramanujan's elliptic perimeter is also calculated.

Perimeter caclulator

Major and minor axes a : b :

Ramanujan's approximation to the perimeter of an ellipse p :

Download perimeter-0.2.js — source code
Example of more accurate values by WolframAlpha
a =  1, b = 1 :  6.2831853071795864769252867665590057683943387987502116419498891846... = 2π (exact)
a =  2, b = 1 :  9.6884482205476761984285031963918294119539183978866008250831163524...
a =  5, b = 1 : 21.010044539689000944699164588473738912894812339134152623096835657...
a = 10, b = 1 : 40.639741801008957425577931011816563791313052134504059403405927819...

APPENDIX — Ramanujan's Perimeter of an Ellipse

$p & = & \pi \left\{(a + b) + \frac{3 (a - b)^2}{10(a + b) + \sqrt{a^2 + 14 ab + b^2}} + \varepsilon \right\}$

REFERENCE

Complete Elliptic Integral of the First Kind — Wolfram MathWorld
Complete Elliptic Integral of the Second Kind — Wolfram MathWorld
Arithmetic-Geometric Mean — Wolfram MathWorld
Mark B. Villarino, "Ramanujan's Perimeter of an Ellipse"
Elliptic integral — Wikipedia

Perimeter caclulator
Major and minor axes	a :	b :
Ramanujan's approximation to the perimeter of an ellipse			p :

Perimeter of an ellips

APPENDIX — Ramanujan's Perimeter of an Ellipse

REFERENCE

SEE ALSO